Grainger CEME

Brian C. Raczkowski with adviser P. W. Sauer

Figure 37: Unsolvable case.

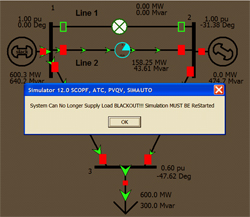
In power system analysis, system loading can be used to determine the margin to a blackout or system collapse. To avoid collapse, methods have been developed to shed load, i.e., disconnect customers from the grid. If shedding load or adding new generation locations is not an option, the system transmission lines need to be changed to ensure customer electricity demands are met. Identifying which line or lines to change is crucial. Figure 37 shows a power system where a line failure or outage brings the system beyond the point of static collapse and insolvability results. The challenge is to know what line or lines in the system contribute to insolvability from a line outage. To bring the system to static collapse, a parameter needs to change. Instead of using a loading change, the discrete event of a line outage is changed to a continuous set of events by increasing the impedance of the line that is being outaged.

Figure 38: (a) LOLC. (b) Line sensitivity.

The example system in Figure 37 is unsolvable for a line 1 outage. Figure 38(a) shows the reactive power loss versus the real power flow during the increase of impedance in line 1. Lines 1 and 4 hit a minimum amount of real power while line 3 hits a maximum. When a minimum or maximum occurs, this is defined to be the line outage loss condition (LOLC). At these minimum and maximum points the sensitivity of real power flow with respect to the change in line outage impedance reaches zero, as shown in Figure 38(b). Using this sensitivity, the maximum real power limit of a line can be changed by changing the appropriate line impedance. The margin to blackout can be increased by this method.

This work is supported by Grainger Fellowships.