A hierarchy of models for distributed energy resources (DERs)
Olaoluwapo Ajala with advisor A.Dominguez-Garcia
As adoption of DERS has accelerated, there is a need to develop comprehensive mathematical tools to model these systems. Models used by control engineers, scientists, mathematicians, and other non-experts in the field of microgrids are required for the analysis and control tasks necessary to develop innovative technologies. For example, to design and test frequency controllers, models that capture phenomena in the same time-scale as the frequency, while neglecting phenomena in faster time-scales, are needed. Although accurate mathematical models can be developed for DERs by utilizing concepts from circuit-theoretic and control theory, these models are often highly complex and too detailed for the particular application. By simplifying them to show less detail, they can represent the phenomena relevant to the application of interest.
Our main contribution is the development of a time-resolution based hierarchy of models for distributed energy resources. Using Kirchhoff’s laws, component terminal relations, and relevant control laws, a high-order model is created. Afterward, reduced-order models are formulated from the high-order model using singular perturbation techniques for model-order reduction. The time resolution for which the reduced-order models is valid is also identified, and all models are explicitly presented with the parameters used for singular perturbation analysis identified. The DER models we developed are used to emulate power systems on the Typhoon HIL device.
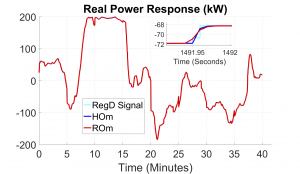
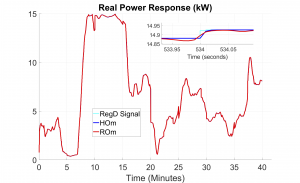
Figures 1 and 2 depict a comparison of a high-order model (HOm) and a reduced-order model (ROm) of two DERs. The model responses to a 40-minute RegD signal are illustrated to show the accuracy of the ROm we developed. This research is supported by DOE